A Greek version of the reserch scientific activities of this topic can be found in odf.pdf format.
OVERVIEW
Monte Carlo methods have been employed in the study of the X-ray absorption and fluorescence properties of medical imaging scintillator detectors. The X-ray properties are studied as a function of the detector's thickness and the incident photon energy or energy spectrum. Several detector materials have been examined such as Gd2O2S, (GOS) Gd2SiO5 (GSO) YAlO3 (YAP), Y3Al5O12 (YAG), LuSiO5 (LSO), LuAlO3 (LuAP) and ZnS. The spectral or monoenergetic photon exposures are modelled in the range from 10 keV to 25 MeV, covering the energies involved in the Medical Imaging Detectors, namely energies in the X-ray Mammography, X-ray Radiography, X-ray Scintigraphy, CT, Nuclear Medicine, PET/SPECT Scanning and Radiotherapy. Modelling and simulation utilise custom-developed MS-Fortran, GNU-gfortran and GNU-c++ codes and codes in EGCnrcMP, MCNP and GATE/GEANT4.
STATE OF THE ART
The scintillator based radiation detectors have been rapidly developed during the last few decades for application in conventional and digital medical imaging such as conventional and digital X-ray radiography or fluoroscopy, X-ray computed tomography, single photon emission tomography (SPECT) and positron emission tomography (PET). In most cases, the scintillator detectors are coupled to optical sensors, such as films, photocathodes, photodiodes, amorphous silicon and thin film transistor technology (a-Si /TFTs), charged coupled devices (CCDs) and complementary metal oxide semiconductors (CMOS) [1-7]. Because of differences in detector design in various medical imaging modalities, and considering the constraints of cost, durability, image quality and patient dose burden, the interest in developing new scintillator materials, exhibiting adequate radiation detection properties, has been renewed [11].
In designing and evaluating scintillator based radiation detectors, it is of importance to determine accurately the radiation detection efficiency of the built-in scintillator detctor. Depending on the imaging application, this efficiency has been expressed by the quantum absorption efficiency or the energy absorption efficiency [8]. Issues, such as the amount of emission and re-absorption of scattered and characteristic X-ray fluorescence radiation, and that of Auger electron energy, are of importance. When scattered or characteristic radiation is re-absorbed within the scintillator detector, apart from the primary interaction point, a loss of spatial resolution and image contrast may occur [8]. On the other hand, if this type of radiation escapes the detector, radiation detection efficiency may be reduced.
Radiation transport phenomena have been extensively studied by application of the Monte Carlo technique. This was proven to be by far the most successful technique for the simulation of the stochastic processes involved in radiation detection [9]. During the last decade, various Monte Carlo simulation packages have become commercially available. Some research groups have reported results on application of such packages in studies of photon transport phenomena in scintillators employed in X-ray medical imaging [8,10,11]. However, the commercially available Monte Carlo simulation packages are of general purpose design [12-15]. Thus, their application is constrained by their expediency and feasibility in specialising to firm situations. In addition, the most popular packages i.e. EGSnrcMP, TART, GEANT and PENELOPE, have been developed and verified for studies mainly in the field of nuclear and high energy physics [12-15].
IMPLEMENTED RESEARCH
Monte Carlo codes have been developed which were applied to the investigation of absorption properties of new and existing scintillator detectors (YAlO3 (YAP), Y3Al5O12 (YAG), LuSiO5 (LSO), LuAlO3 (LuAP) and Gd2SiO5 (GSO) Gd2O2S (GOS), ZnS). Particular interest is paid on the contribution of the generation, re-absorption or escape of K- and L-characteristic photons. The scintillators are modeled and their absorption properties are studied as a function of incident photon energy and scintillator thickness.
Custom-designed Monte Carlo Codes
The Monte Carlo codes are developed using the Microsoft FORTAN Developer PC platform. On 2011 the codes were renewed and ported to GNU gfortran. On 2017, the codes where developed in GNU c++. The codes are designed so as to be efficient for applications in the photon energy range employed in medical X-ray imaging and easily adjustable to various scintillator detector materials.
The photon transport modeling is based on the three processes governing photon interactions in the medical x-ray imaging energy range i.e. coherent- incoherent- scattering and photoelectric absorption. An iterative procedure was used; a photon of certain energy was generated and considered to hit the entrance surface of a scintillator screen of predefined dimensions under known direction angles. Using random numbers and cross-sectional data, the mean-free photon path, as well as the site and type of the subsequent photon interaction were determined. In the case of scatter and by employing a method proposed by Chan and Doi [9,12], based on the use of the form and scatter factors of the materials under study, the photon transport parameters were calculated, i.e. photon energy and direction angles after the interaction and energy transferred to the electrons of the medium. The cross sections of the scintillator materials under study were calculated using the XCOM code, which was based on the tables of Hubbell and Seltzer [17]. The XCOM code was downloaded from the NIST reference database [18]. The form factors and the scatter factors of the scintillator materials under study were calculated from those corresponding to the elements constituting these scintillator materials using specially designed codes. The factors for the elements were also downloaded from the NIST reference database [19]. The iterative procedure was continued until the photon escaped from- or was absorbed within, the scintillator block. In the latter case, all energy of the photon was considered to be transferred to the medium except a part, which was considered to refer to the generation of characteristic fluorescence radiation or Auger electrons. Selection between characteristic fluorescence radiation and Auger electrons was based on a random number generation routine. In the case of characteristic fluorescence radiation simulation, photons were modeled as independent K- or L-characteristic quanta initiating their history at the photoelectric interaction site. The histories of the characteristic photons were followed similarly to those of the primary ones. The characteristic fluorescence photons were generated with azimuthally uniform distribution. In the case of Auger electron production simulation, the part of the energy referring to Auger electrons was considered to be completely transferred to the scintillator block at the site of interaction.
Various Monte Carlo simulation runs are performed. In every run 107 photons were generated and traced. All photon track and energy histories were recorded for further analysis.
Detector parameters studied
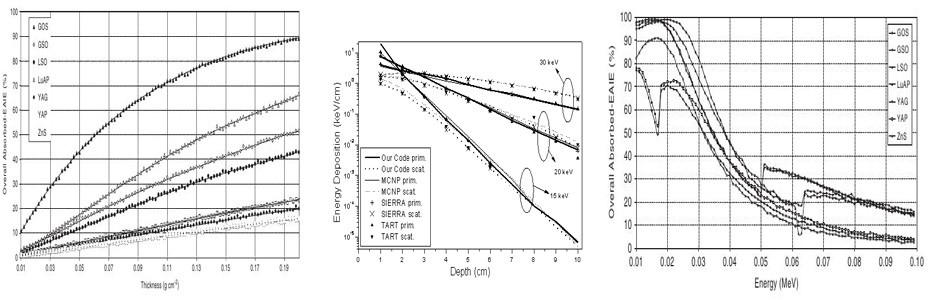
The main detector parameters studied were the efficiency of absorption of incident energy (EAIE) and the quantum absorption efficiency (QAE).
The EAIE of a scintillator is defined as the fraction of the energy of photons absorbed totally within a scintillator block over the total incident energy. EAIE includes all mechanisms of energy deposition within the scintillator’s mass. EAIE is a measure of the absorbed energy and represents the efficiency of a detector to capture the useful x-ray-imaging signal [19-31]. The EAIE is classified into the following classes: (a) Overall Absorbed-EAIE: energy absorbed due to all types of absorption mechanisms i.e. photoelectrons ejected after a photoelectric effect, electrons ejected after a Compton event, and Auger electrons ejected after x-ray fluorescence transitions following a photoelectric effect. This class was accompanied by two additional classes: i) Overall-AF-EAIE: considering that all fluorescence (AF) radiation was completely absorbed within the scintillator and ii) Overall-NF-EAIE: considering that no fluorescence (NF) radiation was absorbed within the scintillator and as a result it escaped. (b) Scattered and Reabsorbed-EAIE: energy absorbed after one or multiple scattering events of the primary photons. (c) Fluorescence Generated-EAIE: energy transferred to characteristic fluorescence quanta following a photoelectric effect. (d) Fluorescence Reabsorbed-EAIE: Fluorescence Generated-EAIE, which was absorbed within the scintillator. Two additional classes related to EAIE are considered: (e) Escaped Incident Energy Fraction: energy escaped from the scintillator block. (f) Fluorescence Escaped-EAIE: the fraction of incident energy corresponding to absorbed photons producing fluorescence radiation escaping the scintillator. In all classes, the energy transferred to the ejected electrons is considered to be fully absorbed at the site of ejection.
QAE is defined as the number of the totally absorbed photons within a scintillator block over the total number of incident photons [29-31]. QAE is accompanied by an additional class: QAE-Photoelectrically Absorbed; via one-hit photoelectrical event. Two additional classes were also considered, corresponding to detected and non detected characteristic fluorescence radiation produced after photoelectric absorption of incident photons: (a) QAE-Fluorescence Forward Escaped; characteristic quanta escaped from the scintillator in the forward direction and (b) QAE-Fluorescence Backward Escaped; characteristic quanta from the scintillator in the backward direction.
The above issues are now (2012) studied with EGSnrcMP and with GATE/GEANT4. New approaches include the simulation of the passage and detection of photons at various scintillator depths. A new optical model has been
Simulated x-ray detector exposures
Modeled scintillators are considered to be exposed to x-rays initiating from a point source located at the central axis of the entrance area of the scintillator block. Photons are generated within a field. Source to scintillator block distance and field size at the entrance surface were defined at the beginning of each Monte Carlo run, together with the energy of the emitted x-ray quanta. The direction angles of the emitted photons were defined by the source to scintillator block distance and the field size dimensions at entrance area, using random numbers. Normal photon incidence is simulated considering zero dimensions of this area. In all cases, normal x-ray incidence was simulated.
REFERENCES
[1] S. Hejazi, D.P.Tauernicht, Med. Phys. 24 (1997) 287
[2] T. Yu, J.M. Sabol, J.A. Seibert, J.M.Boone, Med. Phys. 24 (1997) 279
[3] H.J. Besch, Nucl. Instr. Meth. Phys. Res. A 419 (1998) 201
[4] T.T. Farman, A.G.Farman, J.Digit.Imaging 11 (1998) 169
[5] J.H. Siewerdsen, L.E. Antonuk, Y. el-Mohri, J. Yorkston, W. Huang, V.E. Scarpine, R.A. Street, Med. Phys. 24 (1997) 51
[6] E. Hell, W. Knüpfer, D. Mattern, D., Nucl. Instr. Meth. Phys. Res. A 454 (2000) 40
[7] C.W.E. van Eijk, Phys. Med. Biol. 47 (2002) R85
[8] J.M. Boone, J.A. Seibert, J.M. Sabol, M. Tecotzky, Med.Phys. 26(6) (1999) 905
[9] H. P. Chan, K. Doi, Phys. Med. Biol. 28 (5) (1983) 565
[10] D.A. Jaffray, J.J. Battista, A. Fenster, P. Munro, Med. Phys. 22(7) (1995) 1077
[11] J.M. Bonne, V.N. Cooper, Med. Phys. 27(8) (2000) 1818
[12] GEANT 4 Collaboration, Physics Reference Manual, http://geant4.web.cern.ch/ geant4/G4UsersDocuments/UsersGuides/PhysicsReferenceManual, 2003
[13] E. Dermott, TART 2002, A Coupled Neutron-Photon 3-D, Combinatorial Geometry Time Dependent Monte Carlo Transport Code, Cullen University of California Lawrence Livermore National Laboratory http://www.llnl.gov/cullen1, 2003
[14] F. Salvat, J. M. Fernandez-Varea, J. Sempau, PENELOPE, a code system for Monte Carlo simulation of electron and photon transport, Facultat de Fysica (ECM), Universitat de Barcelona, Spain, 2003
[15] I. Kawrakow, D.W.O. Rogers, The EGSnrc Code System: Monte Carlo Simulation of Electron and Photon Transport, NRCC Report PIRS-701, http://www.irs.inms.nrc.ca/inms/irs/EGSnrc/EGSnrc.html (2003)
[16] H.P. Chan, K. Doi, Med. Phys. 12(2) (1985) 153
[17] J.H. Hubbel, S.M. Seltzer, Tables of X-ray mass attenuation coefficients and mass energy absorption coefficients 1 keV to 20 MeV for elements Z=1 to 92 and 48 additional substances of dosimetric interest. U.S. Department of commerce. NISTIR 5632, 1995
[18] ftp://physics.nist.gov/PhysRefData/
[19] ftp://ftp://www-phys.llnl.gov/pub/rayleigh/RTAB/data_NF/
[20] G. Blasse, B.C. Grabmaier. Luminescent Materials. Springer-Verlag. Berlin, Heidelberg, 1994, p.85
[21] G. Blasse, J. Luminescence. 60&61 (1994) 930
[22] T. Kano, Principal phosphor materials and their optical properties, in S. Shionoya, W.M. Yen (Ed), Phosphor Handbook, CRC Press, Boca Raton, 1999, p.177-186
[23] H. Wieczorek, Rad. Meas. 33 (2001) 541
[24] J. A. Rowlands. Flat Panel Detectors for Digital Radiography, inJ.Beutel, H.L. Kundel, R.L. Van Metter (Eds), Handbook of Medical Imaging, Vol. 1, Physics and Psycophysics, SPIE Press, Bellingham, 2000, pp. 234-248
[25] A.Baciero, L. Placentino, K.J. Mc Carthy, L.R. Barquero, A. Ibarra, and B. Zurro, J. Appl.Phys. 85(9) (1999) 6790
[29] J.M.Boone, X-ray production, Interaction, and Detection in Diagnostic Imaging, in J.Beutel, H.L. Kundel, R.L. Van Metter (Eds), Handbook of Medical Imaging, Vol. 1, Physics and Psycophysics, SPIE Press, Bellingham, 2000, p. 40
[30] I. Kandarakis, D. Cavouras, C. D. Nomicos, G.S. Panayiotakis, Nucl. Instr. Meth. Phys. Res. B 179 (2001) 215
[31] I. Kandarakis, D. Cavouras, Eur. Radiol. 11 (2001) 1083
OTHER RELATED REFERENCES
1.Liaparinos P, Kandarakis I, Cavouras D, Nikolopoulos, D, Valais I.Simulating the emission efficiency and resolution properties of fluorescent screens by Monte Carlo methods, Nuclear Science Symposium Conference Record IEEE CNF 2004; 2:874 – 878
2.Kandarakis I, Cavouras D, Nikolopoulos D, Anastasiou A, Dimitropoulos N , Kalivas N, Ventouras E, Kalatzis I, Nomicos C, Panayiotakis G. Evaluation of ZnS:Cu phosphor as X-ray to light converter under mammographic conditions, Radiat. Meas. 2005; 39:263-275
3.Nikolopoulos D, Kandarakis I, Valais I, Gaitanis A, Cavouras D, Panayiotakis G, Louizi A. X-ray absorption and x-ray fluorescence of medical imaging scintillating screens via application of Monte Carlo methods. Biomed. Tech. 2005; 50(1):1124-1125
4.Tsantilas X, Louizi A, Valais I, Nikolopoulos D, Sakellios N, Karakatsanis N, Loudos G, Nikita K, Malamitsi J, Kandarakis I. Simulation of commercial PET scanners with GATE Monte Carlo simulation package. Biomed. Tech. 2005; 50(1):1124-1125
5.Liaparinos P, Kandarakis I, CavourasD, Nikolopoulos D,Panayiotakis G. Monte Carlo study on radiation absorption properties of Gd2O2S, CsI, LSO, CaWO4 phosphor screens for mammographic x-ray imaging. Europ Radiol 2005; 15(S1): 538-539.
6.Nikolopoulos D, Valais I, Kandarakis I, Cavouras D, Linardatos D, Siannoudis I, Louizi A, Dimitropoulos N, Vattis D., Episkopakis A, Nomicos C, Panayiotakis G. Evaluation of GSO:Ce scintillator in the x-ray energy range from 40 to 140 kV for possible applications in medical X-ray imaging.Nucl.Instr.Method.(A) 2006; 560(2): 577-583
7.Nikolopoulos D, Kandarakis I, Cavourasd, Louizi A, Nomicos C. Investigation of radiation absorption and x-ray fluorescence of medical imaging scintillators by Monte Carlo Methods, Nucl.Instr.Method.(A) 2006; 565:821-832
8.Nikolopoulos D, Kandarakis I, Tsantilas X, Valais I, Cavouras D, Louizi A. Comparative study of the radiation detection efficiency of LSO, LuAP, GSO and YAP scintillators for use in positron emission imaging (PET) via Monte-Carlo Methods, Nucl.Instr.Method.(A) 2006; 569:350-354
9.Valais I, Kandarakis I, Nikolopoulos D, Konstantinidis A, Siannoudis I, Cavouras D, Dimitropoulos N, Nomicos C, Panayiotakis G. Evaluation of light emmission efficiency of LYSO:Ce scintillator under x-ray excitation for possible applications in medical imaging. Nucl.Instr.Method.(A) 2006; 569:201-204
10.Karakatsanis N, Sakellios N, Tsantilas NX, Dikaios N, Tsoumpas C, Lazaro D, Loudos G, Schmidtlein CR, Louizi A, Valais, J Nikolopoulos D, Malamitsi J, Kandarakis J, Nikita K. Comparative evaluation of two commercial PET scanners, ECAT EXACT HR+ and Biograph 2, using GATE, Nucl.Instr.Method (A) 2006; 569: 368-372
11.Gonias P, Bertsekas N, Karakatsanis N, Saatsakis G, Gaitanis A, Nikolopoulos D, Loudos G, Papaspyrou L, Sakellios N, Tsantilas X, Daskalakis A, Liaparinos P, Nikita K, Louizi A, Cavouras D, Kandarakis I, Panayiotakis GS. Validation of a GATE model for the simulation of the Siemens PET Biograph™ 6 scanner Nucl.Instr.Method.(A) 2007; 571(1-2):263-266
12.Nikolopoulos D, Linardatos D, Valais I, Michail C, David S, Gonias P, Bertsekas N, Cavouras D, Louizi A, Kandarakis I. Monte Carlo Validation in the Diagnostic Radiology Range, Nucl.Instr.Method.(A) 2007; 571: 267-269.
13.Nikolopoulos D, Kalyvas N, Valais I, Argyriou X, Vlamakis E, Sevvos T, Kandarakis I. A semi-empirical Monte Carlo based model of the Detector Optical Gain of Nuclear Imaging scintillators. JINST 2012; 7 P1102.
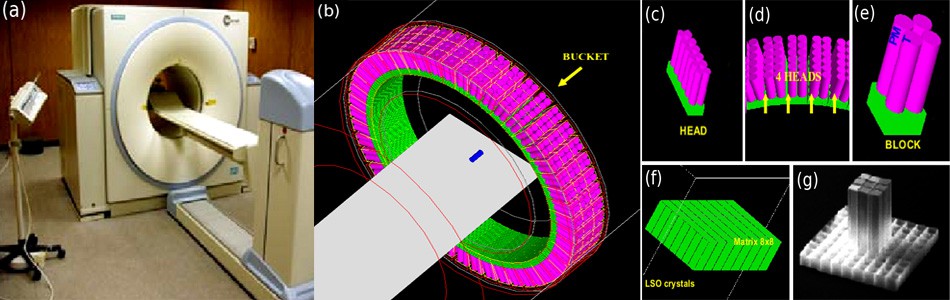
14.Nikolopoulos D, Kottou S, Chatzisavvas N, Argyriou X, Vlamakis E, Yannakopoulos P, Louizi A., A GATE Simulation Study of the Siemens Biograph DUO PET/CT System. Open Journal of Radiology 2013; 3(2):56-65.
15.Nikolopoulos D, Michail C, Valais I, Yannakopoulos P, Kottou S, Karpetas G, Panayiotakis G. GATE Simulation of the Biograph 2 PET/CT Scanner. J Nucl Med Radiat Ther 2014; 5(5):1-6.
16.Nikolopoulos D, Valais I, Michail C, Kottou S, Chatzisavvas N, Yannakopoulos P, Malaxianakis V. Modelling Biograph 2 PET/CT Scanner with GATE. Physica Medica 2014; 30:e94.